

For example, as the Earth's rotational velocity is 465 m/s at the equator, a rocket launched tangentially from the Earth's equator to the east requires an initial velocity of about 10.735 km/s relative to the moving surface at the point of launch to escape whereas a rocket launched tangentially from the Earth's equator to the west requires an initial velocity of about 11.665 km/s relative to that moving surface. The escape velocity relative to the surface of a rotating body depends on direction in which the escaping body travels. Note that this escape velocity is relative to a non-rotating frame of reference, not relative to the moving surface of the planet or moon, as explained below. Note that the minimum escape velocity assumes that there is no friction (e.g., atmospheric drag), which would increase the required instantaneous velocity to escape the gravitational influence, and that there will be no future acceleration or extraneous deceleration (for example from thrust or from gravity of other bodies), which would change the required instantaneous velocity.Įscape speed at a distance d from the center of a spherically symmetric primary body (such as a star or a planet) with mass M is given by the formula v e = 2 G M d = 2 g d Speeds higher than escape velocity retain a positive speed at infinite distance. In other words, if given escape velocity, the object will move away from the other body, continually slowing, and will asymptotically approach zero speed as the object's distance approaches infinity, never to come back. Once escape velocity is achieved, no further impulse need be applied for it to continue in its escape. With escape velocity in a direction pointing away from the ground of a massive body, the object will move away from the body, slowing forever and approaching, but never reaching, zero speed. More generally, escape velocity is the speed at which the sum of an object's kinetic energy and its gravitational potential energy is equal to zero an object which has achieved escape velocity is neither on the surface, nor in a closed orbit (of any radius). It can achieve escape at any speed, given sufficient propellant to provide new acceleration to the rocket to counter gravity's deceleration and thus maintain its speed. The escape speed thus depends on how far the object has already traveled, and its calculation at a given distance takes into account that without new acceleration it will slow down as it travels-due to the massive body's gravity-but it will never quite slow to a stop.Ī rocket, continuously accelerated by its exhaust, can escape without ever reaching escape speed, since it continues to add kinetic energy from its engines. Although the term "escape velocity" is common, it is more accurately described as a speed than a velocity because it is independent of direction the escape speed increases with the mass of the primary body and decreases with the distance from the primary body. It is typically stated as an ideal speed, ignoring atmospheric friction. The magnitude of the force that Earth exerts on us at a fixed place on the surface is determined by the combined effect of gravity and the centrifugal force caused by Earth's rotation.In celestial mechanics, escape velocity or escape speed is the minimum speed needed for a free, non- propelled object to escape from the gravitational influence of a primary body, thus reaching an infinite distance from it. But how large is this acceleration? Well, this depends on the magnitude of the force that Earth pulls us with. This means that if there were no air friction, any two objects falling from the same height would always reach the floor simultaneously. Regardless of the masses or compositions, all bodies accelerate at the same rate in a vacuum.
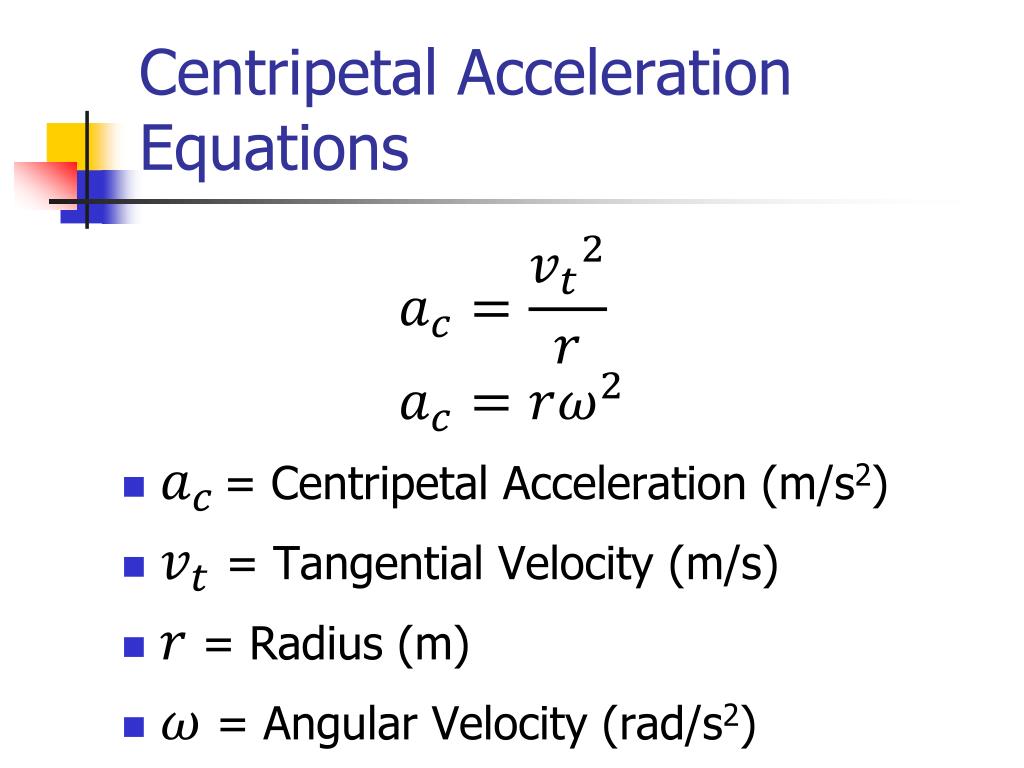
Conservative Forces and Potential Energy.Total Internal Reflection in Optical Fibre.Newton’s and Huygens’ Theories of Light.Einstein's Theory of Special Relativity.Centripetal Acceleration and Centripetal Force.Connecting Linear and Rotational Motion.Electromagnetic Radiation and Quantum Phenomena.Galileo's Leaning Tower of Pisa Experiment.Mechanical Energy in Simple Harmonic Motion.Kinetic Energy in Simple Harmonic Motion.Displacement, Time and Average Velocity.Magnetic Flux and Magnetic Flux Linkage.Potential Energy and Energy Conservation.Conservative and Non Conservative Forces.


 0 kommentar(er)
0 kommentar(er)
